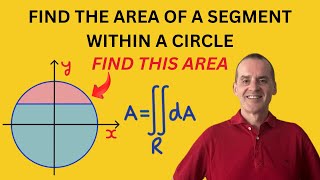
How to find the Area of a segment in a circle using double integration over a polar region

In this video I take a look at how to find the area of a segment in a circle using double integration over a polar region.

In the video I explain how to convert the equation of the circle to polar coordinates and how to convert the line that cuts it, creating the segment, into polar coordinates. So I convert the (x ,y) coordinates to (r, Θ) polar coordinates.

I then take a detailed look at the process of integration to sum the infinitesimally small sectors of area in the radial distance (r) direction to determine the inner integral and then demonstrate how to find the limits of integration in the same direction.

I then move on to taking a detailed look at the outer integral and how to determine the angles that Θ rotates through which provide the limits of integration of the outer integral.

Once the region has been defined I then demonstrate how to evaluate the integrals.

See the video below for more details on polar coordinates
https://youtu.be/IlTqx3La1UU