Numerical Simulation of Ordinary Differential Equations: Integrating ODEs

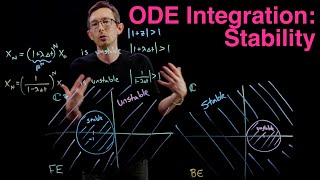
In this video, I provide an overview of how to numerically integrate solutions of ordinary differential equations (ODEs). These solutions are known as trajectories, and provide a powerful computational tool for us to analyze nonlinear systems, where simple analytic techniques may not work. In particular, we only have general solution techniques for linear systems, and for generic nonlinear dynamics, we often are forced to study them numerically using these integration techniques.

Playlist: https://www.youtube.com/playlist?list=PLMrJAkhIeNNTYaOnVI3QpH7jgULnAmvPA
Course Website: http://faculty.washington.edu/sbrunton/me564/

@eigensteve on Twitter
eigensteve.com
databookuw.com

This video was produced at the University of Washington

%%% CHAPTERS %%%
0:00 Problem setup: Integration through a vector field
5:41 Numerical integration to generate a trajectory
15:12 Vector fields may be solution to PDE
18:15 Deriving forward Euler integration