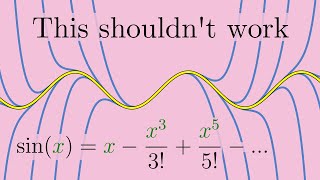Does this infinite series converge? (I solved it!)

We will look at a particular infinite series involving the sine function. We will determine whether the series converges or diverges. The solution involves rational approximations of the number π.

Having some knowledge of trigonometry and infinite series will be useful while watching this video.

Timestamps
00:00 Introduction
08:25 Tame Numbers versus Wild Numbers
11:34 Controlling the Wild Numbers
19:18 Mahler's Theorem
23:59 Conclusion

Correction:
00:40 The digits of pi are written incorrectly. The sequence 2643383279 was inadvertently repeated.

Ravi B. Boppana (2020), "Convergence of a sinusoidal infinite series from Borwein, Bailey, and Girgensohn", https://arxiv.org/abs/2007.11017.

Ravi B. Boppana (2005). Posts by user Ravi B on the thread “Tough infinite series” on the Art of Problem Solving forum.
https://artofproblemsolving.com/community/c7h22093.

Kurt Mahler (1953), "On the approximation of π", Indagationes Mathematicae (Proceedings), 56, 30–42.

Gregory V. Chudnovsky (1982), "Hermite–Pade approximations to exponential functions and elementary estimates of the measure of irrationality of π", in The Riemann Problem, Complete Integrability and Arithmetic Applications, pages 299-322. Lecture Notes in Mathematics 925.

Doron Zeilberger and Wadim Zudilin (2020), "The irrationality measure of π is at most 7.103205334137 . . . ", https://arxiv.org/abs/1912.06345.

Jonathan M. Borwein, David H. Bailey, and Roland Girgensohn (2004), Experimentation in Mathematics: Computational Paths to Discovery. CRC Press.

Wikipedia article on the root test and other standard convergence tests:
https://en.wikipedia.org/wiki/Convergence_tests

Photo of Kurt Mahler from 1970 due to Konrad Jacobs.

To stay up to date, subscribe to this YouTube channel. Thanks!